Highlights
-
This review focuses on recent development of the piezo-electro-chemical coupling multiple systems based on various piezoelectric materials.
-
Comparison of operating conditions and their electro-chemical performance is provided.
-
Challenges, potential future directions, and applications for the development of piezo-electro-chemical hybrid systems are described.
Abstract
Piezoelectric materials have been analyzed for over 100 years, due to their ability to convert mechanical vibrations into electric charge or electric fields into a mechanical strain for sensor, energy harvesting, and actuator applications. A more recent development is the coupling of piezoelectricity and electro-chemistry, termed piezo-electro-chemistry, whereby the piezoelectrically induced electric charge or voltage under a mechanical stress can influence electro-chemical reactions. There is growing interest in such coupled systems, with a corresponding growth in the number of associated publications and patents. This review focuses on recent development of the piezo-electro-chemical coupling multiple systems based on various piezoelectric materials. It provides an overview of the basic characteristics of piezoelectric materials and comparison of operating conditions and their overall electro-chemical performance. The reported piezo-electro-chemical mechanisms are examined in detail. Comparisons are made between the ranges of material morphologies employed, and typical operating conditions are discussed. In addition, potential future directions and applications for the development of piezo-electro-chemical hybrid systems are described. This review provides a comprehensive overview of recent studies on how piezoelectric materials and devices have been applied to control electro-chemical processes, with an aim to inspire and direct future efforts in this emerging research field.
Similar content being viewed by others
1 Introduction
Piezoelectricity was first discovered by P. Cure and J. Curie in 1880 based on their observations of the production of an electrical charge when specific materials were subjected to a mechanical force [1]. The term ‘piezoelectricity’ originates from ‘piezo’ and ‘electricity,’ where ‘piezo’ represents the application of a pressure and ‘electricity’ corresponds to moving electrons [2]. Materials that exhibit piezoelectricity are termed piezoelectric materials, which generate an electric charge in response to applied stress (the direct piezoelectric effect), and develop a mechanical strain when subjected to an applied electric field (the converse piezoelectric effect) [3,4,5,6,7].
The origin of piezoelectricity is related to a non-centrosymmetric distribution of positive and negative electric charges in the unit cell of a material [8, 9]. When a piezoelectric material is subjected to an applied stress or mechanical vibration, the induced displacement of ions results in a net electric charge due to a change in the dipole moment of the unit cell, which builds a piezoelectric potential across the material [10, 11]. Generally, among the 21 crystal point groups of non-centrosymmetric crystals, there are 20 point groups of crystals possessing piezoelectricity, where 10 point groups belong to nonpolar crystals which show piezoelectricity and the other 10 point groups of polar crystals exhibit piezoelectricity and ferroelectricity [8, 9]. Piezoelectric materials belonging to nonpolar crystals which are non-ferroelectric can have no electric net dipole in the zero-stress state and only generate an electric dipole under stress due to the separation of electric charge centers and a resulting induced piezoelectric potential; a good example of such a material is quartz [12,13,14,15,16,17,18]. There are also piezoelectric materials belonging to polar crystals that exhibit a spontaneous polarization in the zero-stress state or no electric field state since there is a separation between positive and negative charges [19, 20]. A good example of such a material is zinc oxide. A subclass of piezoelectric materials are ferroelectric materials belonging to polar crystals, whose spontaneous polarization can be changed permanently and switched when exposed to an external strong electric field, for example, in barium titanate [21, 22]. Since the polarization of a ferroelectric changes with stress, all ferroelectric materials exhibit piezoelectricity by default [11, 23, 24].
Irrespective of the mechanism by which polarization is induced, whether spontaneously or mechanically, the induced electric field across material affects its electrical properties dramatically on the interior and the exterior regions of the material, where the built-in electric field can disrupt electronic energy states, and electric charges are rearranged [25, 26]. If the outside surface of the material is in contact with a medium, the rearrangement of electric charges can alter the electric conductivity, which is highly dependent on the density of electric charge as well as the continuity of the occupiable electronic energy states between the material and the medium [27]. We will see in the review that this process can have a particularly strong influence on electro-chemistry.
Typically, an electro-chemical reaction is driven by an external power source [28,29,30], and the coupling of power generation with electro-chemical process remains a vibrant topic. Large-scale renewable and clean power generation approaches are being considered that store solar and wind energy and subsequently convert it into electrical power for driving electro-chemistry [31,32,33,34]. However, smaller-scale and more local energy, such as mechanical energy in the range of microwatt to milliwatt, can be harvested and utilized by systems based on piezoelectric materials [19, 35]. In recent decades, piezoelectrically induced electric fields have been used to control catalytic rates in chemical solutions [36,37,38], the corrosion rate of metals in etchant solutions [39,40,41,42,43], self-charging systems [44,45,46,47,48,49,50], and a variety of other electro-chemical processes [51,52,53]. The coupling of piezoelectricity and electro-chemistry is termed piezo-electro-chemistry, where a piezoelectrically induced electric charge or potential difference generated by a mechanical stress can influence electro-chemical reaction systems [54,55,56].
There are a variety of excellent reviews on electro-chemistry [19, 57,58,59,60,61,62,63,64], but those that specifically focus on piezoelectrically influenced electro-chemical reactions have received limited attention to date. This review places a focus on the range of piezoelectric materials used for controlling electro-chemical processes. It will provide an overview of the basic characteristics of piezoelectric materials and comparison of the operating conditions and electro-chemical performance. The reported piezo-electro-chemical mechanisms will then be examined in detail. Within this review, we have collected virtually all published research work to date on the use of piezoelectric materials for controlling electro-chemistry; this body of work is summarized in Table 1 which contains detailed information regarding the specific piezoelectric materials, along with the electro-chemical processes and performance. In addition, the piezo-electro-chemical reaction systems to be covered within this review include materials that are in bulk [65, 66], fiber [67,68,69], sheet [70, 71], flower [37, 72, 73], particle [74, 75], and irregular [32, 76] form. The piezoelectric materials include ferroelectric perovskites [77, 78], wurtzite zinc oxide [79, 80], two-dimensional layered transition metal dichalcogenide-based materials [81, 82], organic piezoelectric materials [44, 83, 84], and biological materials [85] that are used for a variety of applications such as selective deposition [38, 77, 86], hydrogen production [32, 65, 69], dye degradation [73, 76, 87,88,89], self-charging power cells [44, 45, 49, 83], and others [47, 90]. The above-mentioned piezo-electro-chemical reactions are shown schematically in Fig. 1, and the intention of this review is to overview recent studies on piezoelectric materials and devices that have been applied to control electro-chemical processes and inspire increasing efforts in this new and emerging research field.
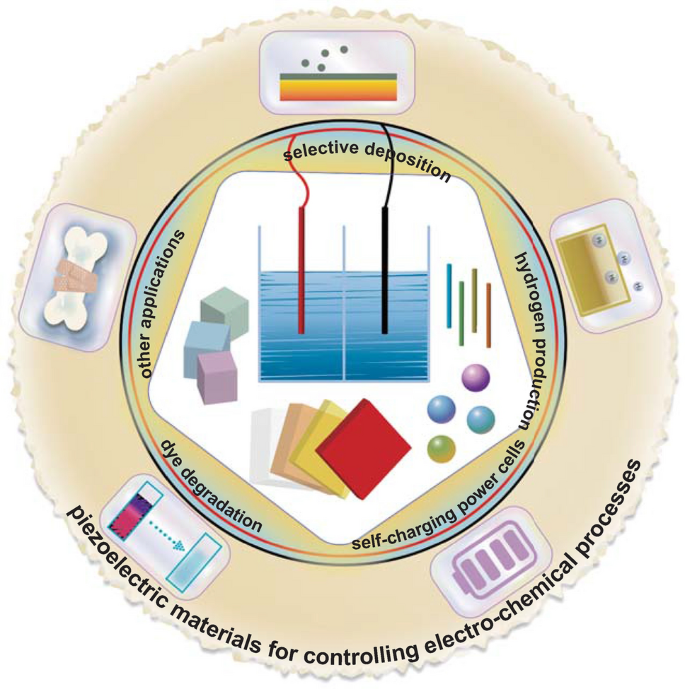
Piezo-electro-chemical reaction systems to be covered in the review with various materials and practical applications
2 Mechanism of Piezoelectric Controlled Electro-chemistry
2.1 Fundamental Electro-chemical Mechanism
The piezo-electro-chemical processes, which involve the coupling of piezoelectricity and electro-chemistry, are chemical reactions driven by piezoelectrically induced electric charge and voltage. The fundamental quantitative relationships in electro-chemistry can be concluded as the well-known law of ‘Faraday’s laws of electrolysis,’ published by Faraday in 1834 [91]. During a typical electro-chemical reaction, the mass of produced material (m) is related to the total transferred electric charge (Q), which can be summarized by:
where M and z, related to specific materials, represent the molar mass of the substance in grams per mol and the valence number of ions of the substance (individual electric charges transferred per ion), respectively. In addition, F is known as the Faraday constant with a fixed value of 96,485.3 C mol−1. For a specific electro-chemical reaction system, it can be seen that M, F, and z are constant, so that the larger the value of Q the larger the mass of produced material, m.
For piezo-electro-chemistry, the magnitude of Q is primarily a response to the charge output of the specific piezoelectric materials as a result of a change in polarization under a mechanical load. The material factors for output will be discussed in the following section.
2.2 Piezoelectric Material Factors
Piezoelectric material factors that influence the value of Q can be firstly related to aspects of the most suitable structure, since materials of the same nature and different structures have a far-reaching effect on the transfer of electric charges or ions. A range of structures have been suggested as a piezo-separator for self-charging power cells. For example, the migration rate of lithium ions can be evaluated by an important parameter, the ionic conductivity, and this materials parameter in the solid state refers to the ease of ion motion in a crystal lattice. Porous nanostructured PVDF films have shown higher ionic conductivity compared to a quasi-bulk film, and the reported explanation of this phenomenon is that the pores can act as a pathway for Li ions to move across the piezo-separator solid [83, 84]. Additionally, porous structures are beneficial for a higher intake of electrolyte solution to facilitate the migration of lithium ions. Therefore, the design of piezoelectric material structures should take account of the influence in the transfer of ions and electric charges.
In addition, in order to ensure efficient ion or electric charge transfer to surrounding atoms/molecules that participate in electro-chemical oxidation–reduction processes, the selected material requires an enhancement of the specific surface area and reactivity [91]. On varying the shape and size, especially at the smaller scale, Li et al. observed a peak shift and an intensity change of the peaks for Raman spectra of ferroelectric BFO materials due to changed spin–phonon coupling and lattice distortions [92], and a shift in absorption edge for UV–Vis absorption spectra of differing BFO samples which depended on varying crystal field intensity [93]. Thus, the optical absorption properties of materials can be strongly influenced by variations in the crystal structure including shape and size and play a significant role in electro-chemical reactions affected by light illumination; the influence of illumination on a piezo-electro-chemical reaction system will be discussed in the following section. In all, there are a variety of shape- and size-controlled physical/chemical factors that are related to mass transfer, contact area, bonding interactions as well as local crystal structure change, where factors mentioned here are generally issues of morphology, and more piezoelectric-related details will be described in the following paragraphs.
The practical output performance of piezoelectric materials closely relates to piezoelectricity, a linear electromechanical coupling, which can be considered as the following equation:
where Pi, dijk, and σjk represent the polarization vector, the piezoelectric third-rank tensor, and the stress tensor, respectively [94]. The piezoelectric third-rank tensor dijk comprises a piezoelectric matrix with typical values dependent on the specific crystalline structure. During a typical piezo-electro-chemical process, the piezoelectrically induced open-circuit voltage (Vi) follows the rule of piezoelectricity [27]:
where w is the material size, ε0—is the vacuum permittivity, and εr is the relative permittivity. In addition, dijk is always considered as the piezoelectric charge sensitivity coefficient or piezoelectric constant in the pC/N or pm/V range [95, 96]. On the basis of Eqs. (2) and (3), the piezoelectrically induced output depends on the piezoelectric constant, the permittivity, the size, and the applied stress for a typical material.
The loading stress for a specific material can be related to the shape of the material [78, 97,98,99]. A larger and easier level of deformation can be achieved in piezoelectric materials with a higher aspect ratio, which induces a higher electrical charge generation [100]. Therefore, the piezoelectric output of materials in one or two dimensions such as nanofibers (nanowires or nanorods) and nanoflowers is often larger than the equal-size spherical particles’, because of the nature of its large and easy deformation [67, 97]. Taking issues of material shape and size into consideration, the piezoelectric potential distribution of nanowires and nanoparticles was simulated via a finite element method with COMSOL multiphysics software, as shown in Fig. 2 [101]. Individual BTO nanowires oriented along the z-axis are strained by a point-applied lateral force, face-applied axial compression, and face-applied lateral pressure in Fig. 2a–c. For contrast, a BTO nanoparticle with a quadrilateral shape indicates the piezoelectric potential is proportional to the applied pressure and the size of nanoparticle, as in Eq. (3). When the applied pressure is 108 Pa, BTO nanowire and nanoparticle of the same size of 100 nm exhibit distinctly different voltage outputs: 11.2 V for the nanowire and only 1.1 V for a nanoparticle due to higher and easier deformation of the nanowire. These simulation results are consistent with the actual phenomena of observations, where the practical degradation rate constant k is in the order: knanowire > knanoparticle [101].
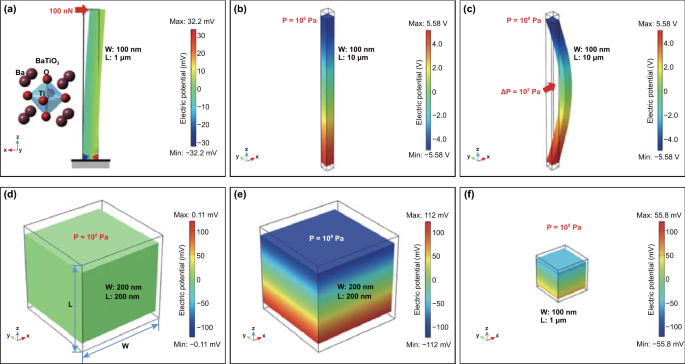
a A BTO nanowire with a diameter of 100 nm and a length of 1 μm stressed on the top by a lateral force with 100 nN where the bottom side is grounded and fixed. b A BTO nanowire under an axial compression with the pressure of 108 Pa. c A BTO nanowire stressed at middle part under a lateral deformation with the pressure of 108 Pa. A BTO nanoparticle with a size of 200 nm under the pressure of d 105 Pa and e 108 Pa. f A BTO nanoparticle with a size of 100 nm under the pressure of 108 Pa. Reproduced with permission [101]. Copyright 2018, Elsevier
In addition, the loading stress created by external forces or mechanical vibrations can induce a forced oscillation of the material. When an oscillating force is applied at the resonant frequency of a material/structure, it will oscillate at a higher amplitude compared to non-resonant frequencies, where a resonant frequency is in harmonic proportion to a natural frequency of material. Therefore, a larger deformation can be achieved in materials forced at its resonant frequency. A variation in the geometry, size, crystalline structure, and atomic composition can influence the fundamental mechanical properties of materials. Here, we consider materials under an elastic deformation, which obey the rule of resonance, as follows [102]:
Equation (4) describes the resonance frequency (f r) of a typical rod vibrated longitudinally, where l, E, and ρ are the length, Young’s modulus, and density of the rod material, respectively. In addition, E is one of mechanical properties that define the relationship between stress and strain.
Equation (5) is based on the same rod material vibrated by a lateral oscillation, where I and S represent the axial moment of inertia and cross-sectional area, respectively.
Equation (6) provides the value of f r that is dependent on a rectangular sheet with a thickness of H and an area size of a × b, where σ is Poisson’s ratio that equals to the negative of the ratio of transverse strain to axial strain. For the resonance equations above, when the values of both x = y = 1, the resonance frequency f r is the first-order resonance frequency, which can be considered as the natural frequency of the material.
According to Eqs. (4)–(6), the dependence of the resonant frequency on its vibration mode, geometry, size, and fundamental mechanical properties can be determined. A variety of piezoelectric materials show a different response to an applied mechanical force or vibration, ranging from low frequency to high frequency, so that the selection and design of piezoelectric materials or related hybrid systems can be optimized to match practical applications with a specific frequency band spectrum. As reported, the use of hydrothermally synthesized BFO square sheets for piezo-electro-chemical hydrogen production exhibits an enhanced production rate when subjected to mechanical vibrations at a frequency near their natural frequency (~ 45 kHz) compared to other frequencies [71]. For further practical applications, oceans provide a wide range of vibration energy sources with a frequency band ranging in 10 to 500 Hz for seismic exploration and commercial shipping, 500 Hz to 500 kHz for small vessel sonar, and sea-surface agitation, and > 25 kHz for thermal noise [103,104,105,106,107].
In this section, we have discussed the dependence of piezoelectric materials characteristics on the output performance, where the shape, size, and mechanical properties have been taken into detailed consideration. We now discuss the charge transfer mechanism for piezo-electro-chemical and piezo-photo-electro-chemical processes.
2.3 Charge Carrier Separation and Transfer
In addition to the geometry, size, and mechanical properties of the piezoelectric materials affecting the piezoelectric output, the applied experimental conditions are also of importance for piezo-electro-chemical processes. To induce piezoelectricity, mechanical vibrations with a specific orientation and amplitude can affect the emergence of electric carriers [19, 108]. In addition, illumination by light leads to excited photo-electro-chemical reactions in piezoelectric materials that are affected by the built-in piezoelectric potential of the material [38, 109].
During a typical piezo-electro-chemical reaction, the charge transfer mechanism can be described by the following. When there is no externally applied mechanical force on the piezoelectric material, it remains at equilibrium, with occupiable electronic states and the surface energy bands in quasi-static states. When subjected to only mechanical vibrations, piezoelectricity leads to a change of polarization, which can develop a piezoelectrically induced electric field across the piezoelectric, thereby leading to electric charge carriers reorienting across different ends of the material. Consequently, both the occupiable electronic states and the surface energy bands are affected by the accumulation of these electric charges on the different sides of the material [110, 111]. Of particular note is that the temperature of the reaction system must be over 0 K; as a result, separated electron–hole pairs in such a piezoelectric semiconductor can be thermally activated. The above-mentioned occupiable electronic states and bending energy bands guide the thermally activated separated electron–hole pairs to the surface of the material, which can participate in oxidation and reduction reaction to generate active species for electro-chemical processes [37]. When the accumulated electric charges on the material surface counteract the built-in electric field, the system returns to an equilibrium level. The reverse change of polarization results in reverse accumulation of electric charges and reverse transfer of electron–hole pairs.
A schematic of this piezoelectrically induced charge transfer mechanism is shown in Fig. 3a, which is a system that is stimulated by mechanical vibration only [37]. The variation of the orientation and magnitude of polarization electric field across the material depends on the material type (ferroelectric or piezoelectric), and the force as a function of time since both varying the direction of vibration and mechanical intensity can influence the polarization field. Starr and Wang have pointed out the difference between the three subcategories of materials in terms of their polarization and electric dipoles [3]. In the absence of strain, ferroelectric materials exhibit a spontaneous polarization, where positive and negative electric charge centers exhibit no superposition, giving rise to resultant electric dipoles along the material, while piezoelectric materials without ferroelectricity such as quartz exhibit a zero internal dipole. However, upon the application of a strain both polarization orientation and magnitude can be varied for piezoelectric materials, since there is a separation between the positive and negative electric charge centers, where the polarization orientation is related to the direction of applied force in general.
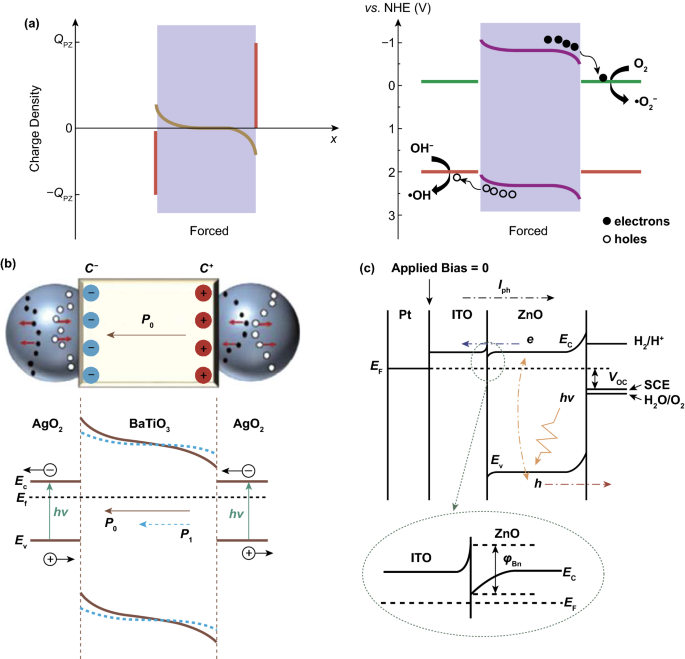
a Schematic of the piezo-catalytic effect in BTO–PDMS. Reproduced with permission [37]. Copyright 2019, American Chemical Society. b Schematic of charge carrier separation and transfer in Ag2O nanoparticles attached to two opposite surfaces of a BTO nanocube. Reproduced with permission [36]. Copyright 2015, American Chemical Society. c Schematic illustration of the band lineup of the entire PEC system. Reproduced with permission [116]. Copyright 2011, American Chemical Society
For individual photo-electro-chemical process in a semiconductor, as is well known, the absorption activity is determined by the band gap, which is relevant to the electronic energy states [112]. For a typical semiconductor with a direct band gap which is suitable for the separation of electron–hole pairs, the general absorption coefficient near the band edge obeys the Tauc equation [93, 113]:
where α, h, ν, A, and Eg are the absorption coefficient, Planck’s constant, irradiation frequency, proportionality constant, and energy band gap, respectively. According to Eq. (7), the adsorption activity is relevant to irradiated light and the material’s Eg. Generally, irradiated light sources with a different wavelength and intensity throughout the reaction can be controlled precisely; however, the value of Eg is also related to changing spin–phonon coupling and lattice distortions which is influenced by shape and size. A high absorption coefficient is preferred for optical absorption during the photo-electro-chemical reaction and demands that the optical absorption of a material’s characteristic wavelength corresponds to the value of Eg within in the solar spectrum. In addition, the selection of material’s Eg should also take the challenge of charge separation into consideration.
In order to achieve efficient photo-electro-chemical reactions, research effort has been focused on impurity doping, increasing the reaction temperature, and the construction of heterojunction structures to restrain the recombination of photo-generated electron–hole pairs [114, 115]. When piezoelectric materials participate in photo-electro-chemical reactions, the piezoelectric polarization acts as an adjustment for the barrier height of the semiconductor at the interface, where electronic energy states can be influenced strongly. Two examples of electric charge transfer mechanism for the hybrid piezo-photo-electro-chemistry system are now described. One example is based on Ag2O–BTO hybrid nanostructures, where a schematic of the charge carrier transfer mechanism is shown in Fig. 3b [36]. To summarize the Ag2O–BTO hybrid system in brief, the Ag2O acts as a semiconductor to produce photo-generated carriers, while the ferroelectric polarization of BTO accelerates the separation of electron–hole pairs. Under dark conditions, there are limited electron and hole electric carriers in the Ag2O. When excited by photons, charge carriers generated in the Ag2O nanoparticles are attached on two sides of the BTO nanocube; however, electrons and holes often exhibit high rates of recombination. An electric field is built across BTO along its spontaneous polarization orientation, which provides a driving force for attracting electrons and holes moving to opposite sides, thereby reducing recombination. The separation of electron–hole pairs continues until all of the piezoelectric polarization charges are fully screened. When subjected to a compressive stress, the polarization potential is diminished, indicating the screened charges can be released, which is a fast discharge process. Subsequently, the recovery of deformation reconstructs the balance between the screened charges and the built-in field, which is a recharging process.
Another example is based on a ITO/ZnO heterojunction structure, where both ITO and ZnO are n-type semiconductors, but the free charge carrier concentration of ZnO is much lower [116]. Figure 3c demonstrates the band lineup of the photo-electro-chemical reaction system under no illumination or external bias. At the interface between ITO and ZnO, a Schottky barrier-like n–n junction is formed with a small barrier (φBn) due to the larger work function of ZnO. In addition, the depletion region near the ZnO is much wider than that near ITO since the free charge carrier concentration of ZnO is much lower than ITO. When subjected to illumination, photo-generated separated electron–hole pairs move through the interface between ZnO and ITO and eventually reach the electrode and electrolyte to participate in electro-chemical oxidation–reduction reactions. Therefore, the heterojunction barrier φBn between piezoelectric semiconductor and electrode becomes a significant obstacle for charge transfer. Additionally, when a piezoelectric semiconductor ZnO is subjected to a tensile strain, the energy bands near the electrolyte increase, where the valence band (VB) is closer to the oxidation potential (Eox). Thus, holes are sufficiently active to drive the oxidation process, and electrons on the conductor band (CB) drift to the ITO side due to the increase of the energy band near the electrolyte interface. Meanwhile, the energy bands of ZnO decrease near the ITO region, and φBn decreases as a result that can benefit charge transfer. When the piezoelectric semiconductor ZnO is subjected to a compressive strain, the energy bands near the interface of ZnO and ITO increase, while those near the interface of ZnO and electrolyte decrease. As a result, there is an increase in φBn, so that the separation and transfer of the photo-generated electric charge carriers show a further restrain, which prevents the progress of the electro-chemical reactions.
2.4 Electro-chemical Processes in Specific Applications
In the previous section, we have discussed electro-chemical processes controlled by piezoelectricity. A detailed description and contrast in mechanisms for specific applications will be provided in this section. As mentioned in Sect. 2.1, electro-chemical processes for practical applications obey Faraday’s law of electrolysis. The premise of whether or not a redox reaction can occur is the relationship between the induced piezoelectric output and the oxidation–reduction potentials of the target products. The possible electro-chemical equations in detail for a variety of applications are given in Table 2.
For selective deposition, the characteristic oxidation–reduction potentials of a variety of metal salts lead to various electro-chemical reactions occurring on opposite facets of the piezoelectric particles. Typical selective deposition reactions take place on the surface of piezoelectric BTO in AgNO3 and Pb(C2H3O2) aqueous solutions, where the chemical equations are illustrated as follows [77]:
For piezo-electro-chemical hydrogen evolution, different piezoelectric materials generate a specific voltage when subjected to applied force, and piezoelectric materials can theoretically drive electro-chemical hydrogen production when the piezoelectrically induced potential exceeds the oxidation potential of hydrogen ions (1.23 V), where the fundamental electro-chemical reactions of hydrogen production are as follows [69]:
Here, the mass of produced hydrogen and oxygen is in proportion to the amount of generated electric charge, and a large amount of hydrogen production is preferred, where of great significance is the long lifetime of negative charges actively for hydrogen generation. In order to decrease the recombination of piezoelectrically induced charges and extend the lifetime of negative charges, sodium sulfite (Na2SO3) is a common sacrificial agent which can scavenge positive charges effectively [117]. For piezo-electro-chemical dye degradation, the generation of active radicals is suggested as the necessary species for further decomposing organic dye molecules, such as superoxide (·O2−) and hydroxyl (·OH) radicals [118,119,120]. Brief procedures for piezo-electro-chemical wastewater treatment can be expressed by the following [69]:
The degradation products generally include 2-naphthol, 2-hydroxy-1,4-naphthoquinone, and smaller aromatic intermediates [87, 121,122,123].
The electro-chemical processes in self-charging power cells exhibit a more complex behavior, which can be generally divided into several steps for charging reactions driven by a cyclic compressive strain. If we take a typical self-charging power cell device as an example, as shown in Fig. 4, when the power cell device is subjected to compressive strain, the piezoelectric PVDF separator builds positive and negative potentials at the cathode and anode sides, respectively. Li ions from the cathode move across the piezo-separator film driven by the built-in piezoelectric field. This process can be considered as the charging reactions for the special lithium-ion battery, where the electro-chemical oxidation–reduction reactions occur at the cathode and anode sides as follows [44]:
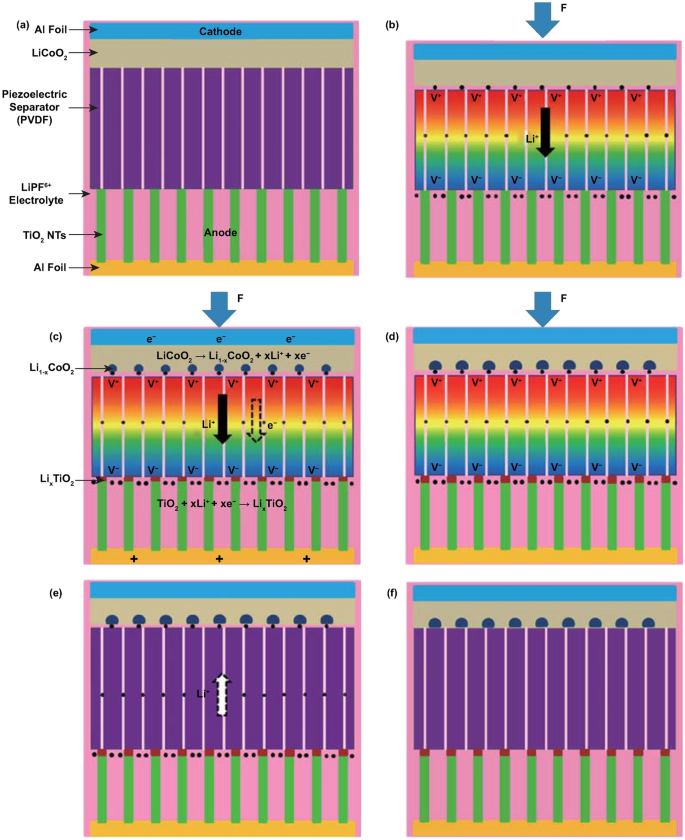
a Schematic of the self-charging power cell in discharged state. b Under compressed stress, piezoelectric PVDF film can create a potential. c Under the piezoelectric field, Li ions are driven to migrate from the cathode toward the anode, which leads to the corresponding charging reactions at the electrodes. d Chemical equilibrium is rebuilt in the self-charging power cell. e When the compressive stress is released, the piezoelectric field across the piezo-separator disappears; in the meanwhile, the Li ions diffuse to the cathode side. f New chemical equilibrium is reached, and one typical cycle of self-charging reaction is accomplished. Reproduced with permission [44]. Copyright 2012, American Chemical Society
Meanwhile, free positive and negative charges dissipate, respectively, at the cathode and anode sides, until an electrostatic equilibrium status is rebuilt. When the compressive strain is released, partial Li ions diffuse back to the cathode, and the as-mentioned electro-chemical reactions process to the inverse sides. Thus, when a cyclic compressive strain is applied, the power cell can realize intermittent self-charging cycles. From the perspective of a chemical reaction, the greatest difference of self-charging power cells with other electro-chemical systems is that the reactions can be controlled to react toward the inverse directions when subjected to appropriate conditions.
In Sect. 2, we overview the reported mechanisms of coupling piezoelectric and electro-chemical effects. In the following section, specific piezoelectric materials applied in electro-chemical processes will be described in detail.




